Flattening the Curve: How You Can Learn to Stay Healthy With STEM
There probably aren’t too many people on the planet that haven’t heard of COVID-19. The viral contagion that caused a global pandemic has taught us a lot about the science and math behind viral transmission, exponential growth, and herd immunity.
Unlike epidemics/pandemics in the past, COVID-19 symptoms aren’t as severe and, thus, not as easy to identify right away. The time it takes before an infected person may show signs can be between two to 14 days. COVID-19 also seems to be more transmissible than past viruses and has spread across the globe quickly as a result.
The World Health Organization and the Centers for Disease Control have recommended social distancing to “flatten the curve” — an effort to reduce the potential for transmitting the virus from one person to another and hopefully keep more people healthy. As a result, we’ve seen schools, organizations and businesses asking their students, employees and participants to work/study from home, if possible. To better understand what “flattening the curve” means, and to explore how epidemics/pandemics can happen, we can use models and simulations.
A mathematical model is a useful tool for describing natural phenomena, such as the epidemiology of the COVID-19 pandemic. Some mathematical models are empirically based, while others are theoretically based. Empirical models are built from after-the-fact observations, for example, fitting a polynomial regression to a set of observed COVID-19 cases. Empirical models are justified solely by how well they extrapolate and predict future values. This type of model has several weaknesses. They are only as good as the data they are based on, there could be odd mathematical behavior outside of the dataset that has no meaning to the phenomenon, and importantly, they lend no insight into the phenomenon.
Alternatively, a theoretical mathematical model is based on a currently accepted theory. Historically, theoretical models have made considerable advances in the sciences. Theoretical models can predict a phenomenon before it is ever observed. Examples include the existence of Neptune, anti-mater, the cosmic microwave background, and the Higgs boson, to name just a few.
Some phenomena have a random component, for example, rolling dice, radioactive decay and evolution. A Monte-Carlo mathematical model is one way to describe and predict this type of phenomenon. The model used in “Flattening the Curve” is a Monte-Carlo simulation. The hectic motion of the particles models the random interactions people could have while shopping at a crowded store.
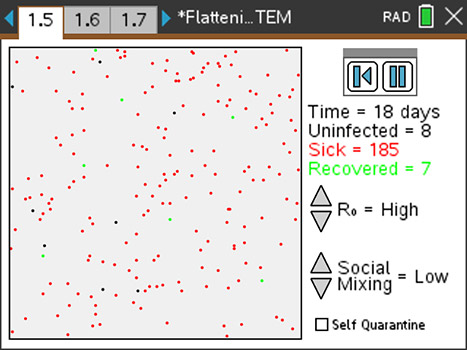
Social distancing, R0, and self-quarantine can be simulated by changing the way the particles interact. Since the “Flattening the Curve” simulation is Monte-Carlo, the data is never precisely the same each time, which is the way a real pandemic spreads.
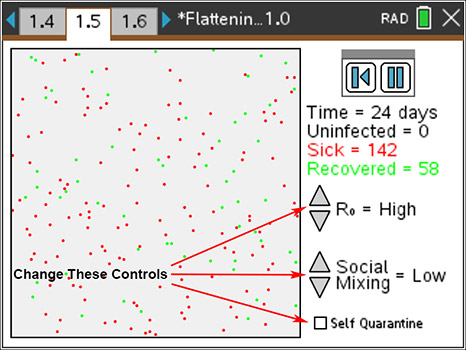
Run the simulation several times using different parameters, and note the effect on the graphs on the following pages.
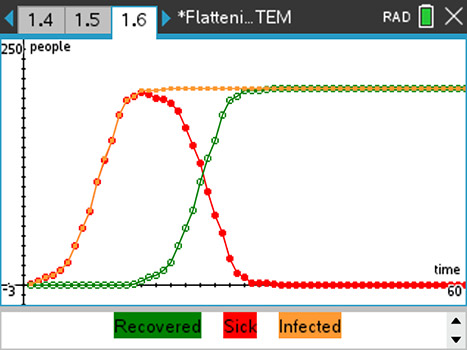
Analyze the data by fitting an empirical model to the simulated data during the exponential phase of growth. What changes in the simulation will flatten this curve?
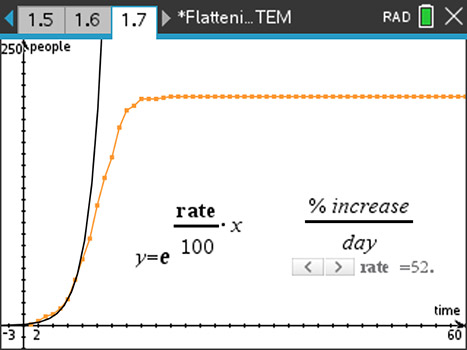
This simulation is a model of a very complicated phenomenon. The model is not complete and is not based on, nor uses, actual COVID-19 case data. This model assumes everyone who is sick will recover; regrettably, this is false. This feature was purposeful to be sensitive, not to be misleading. The intent of the simulation is to have a discussion on how a pandemic spreads and the mathematics of modeling and to also understand how personal behaviors can help to reduce the spread of the disease. You can download the companion file, “Flattening the Curve” for use with the TI-Nspire CX family of products, and help your students learn how to “flatten the curve” and to stay healthy.
About the author: Fred Fotsch is a retired high school science teacher who now works as the STEM education manager at Texas Instruments.
Tagcloud
Archive
- 2025
- 2024
-
2023
- January (3)
- February (3)
- March (5)
- April (3)
- May (3)
- June (3)
- July (2)
-
August (6)
- 5 Ways to Spruce Up Your Classroom for Back to School
- Day of the Dog: Which Dog Is Roundest?
- Women Who Code: A TI Intern’s Fascinating STEM Journey
- 6 Sensational TI Resources to Jump-Start Your School Year
- 3 Back-to-School Math Activities to Reenergize Your Students
- A New School Year — A New You(Tube)!
- September (2)
- October (3)
- November (1)
- 2022
-
2021
- January (2)
- February (3)
- March (5)
-
April (7)
- Top Tips for Tackling the SAT® with the TI-84 Plus CE
- Monday Night Calculus With Steve Kokoska and Tom Dick
- Which TI Calculator for the SAT® and Why?
- Top Tips From a Math Teacher for Taking the Online AP® Exam
- Celebrate National Robotics Week With Supervised Teardowns
- How To Use the TI-84 Plus Family of Graphing Calculators To Succeed on the ACT®
- AP® Statistics: 6 Math Functions You Must Know for the TI-84 Plus
- May (1)
- June (3)
- July (2)
- August (5)
- September (2)
-
October (4)
- Transformation Graphing — the Families of Functions Modular Video Series to the Rescue!
- Top 3 Halloween-Themed Classroom Activities
- In Honor of National Chemistry Week, 5 “Organic” Ways to Incorporate TI Technology Into Chemistry Class
- 5 Spook-tacular Ways to Bring the Halloween “Spirits” Into Your Classroom
- November (4)
- December (1)
-
2020
- January (2)
- February (1)
- March (3)
- April (1)
- May (2)
- July (1)
- August (2)
- September (3)
-
October (7)
- Tips for Teachers in the time of COVID-19
- Top 10 Features of TI-84 Plus for Taking the ACT®
- TI Codes Contest Winners Revealed
- Best of Chemistry Activities for the Fall Semester
- Best of Biology Activities for the Fall Semester
- Best of Physics Activities for the Fall Semester
- Best of Middle Grades Science Activities
- November (1)
- December (2)
- 2019
-
2018
- January (1)
- February (5)
- March (4)
- April (5)
- May (4)
- June (4)
- July (4)
- August (4)
- September (5)
-
October (9)
- Art in Chemistry
- Which Texas Instruments (TI) Calculator for the ACT® and Why?
- Meet TI Teacher of the Month: Jessica Kohout
- Innovation in Biology
- Learning With Your Students
- A first-of-its-kind STEM strategy charts path to help educators
- #NCTMregionals Hartford 2018 Recap
- The Math Behind “Going Viral”
- Real-World Applications of Chemistry
-
November (8)
- Testing Tips: Using Calculators on Class Assessments
- Girls in STEM: A Personal Perspective
- 5 Teachers You Should Be Following on Instagram Right Now
- Meet TI Teacher of the Month: Katie England
- End-of-Marking Period Feedback Is a Two-Way Street
- #NCTMregionals Kansas City 2018 Recap
- Slope: It Shouldn’t Just Be a Formula
- Hit a high note exploring the math behind music
- December (5)
- 2017
- 2016
- 2015
